[最も欲しかった] tan^2x-sec^2x=1 118664-5.25064634

I know that and The next step would then be to say that but now what? Because if you take tan^2(x) 1 = sec^2(x) then tan^2(x) = sec^2(x) 1 And if we input that into the original then sec^2(x) * sin^2(x) sin^2(x) or tan^2(x)Viewed 24k times 1 I am trying to express this problem in terms of sin/cos and simplify I couldn't figure out where to go, I tried as best I could I know the answer is 1 but I am more interested to know how to do this problem tan 2 ⁡ x − sec 2 ⁡

Every Day I M Calculatin I D3 Unit Q Pythagorean Identities

5.25064634

5.25064634-Trigonometry Graph y=sec (2x) y = sec(2x) y = sec ( 2 x) Find the asymptotes Tap for more steps For any y = sec ( x) y = sec ( x), vertical asymptotes occur at x = π 2 n π x = π 2 n π, where n n is an integer Use the basic period for y = s e c ( x) y = s e c ( x), ( π 2, 3 π 2) ( − π 2, 3 π 2), to find the verticalCos(3x) = 4cos^3(x) − 3cos(x) Here are some Important results * Pythagorean Identities sin 2X cos 2X = 1 1 tan 2X = sec 2X 1 cot 2X = csc 2X * Negative Angle Identities sin (X) = – sin X, odd function csc (X) = – csc X, odd function

Chapter 2 Answers

Free integral calculator solve indefinite, definite and multiple integrals with all the steps Type in any integral to get the solution, steps and graphYou could take tan(x) out of the fraction, but I still don't know how to go about simplifying it The book says the answer is Trig Use the fundamental identities to simplify the expression cot beta sec beta I used 1tan^2u=secu since cot is the inverse of tan I flipped the tangent, then so it was 1 (1/tan)Integration of tan^2x sec^2x/ 1tan^6x dx Ask questions, doubts, problems and we will help you=> `1 2*tan^2x` It is seen that `sec^4 x tan^4 x = 1 tan^2 x` is not an identity, instead `sec^4x tan^4x = 1 2*tan^2x

 A few hints 1 sec x = 1/(cos x) 2 (sin x)/(cos x) = tan x That should give you a good start Ex 34, 8 Find the general solution of the equation sec2 2x = 1 – tan 2x sec2 2x = 1 – tan 2x 1 tan2 2x = 1 – tan2x tan2 2x tan2x = 1 – 1 tan2 2x tan2x = 0 tan 2x (tan2x 1) = 0 Hence We know that sec2 x = 1 tan2 x So, sec2 2x = 1 tan2 2x tan 2x = 0 taTrig Identities Nice work!

Tan^2xsec^2x/1tan^6x Ask questions, doubts, problems and we will help youCalculus 2, integral of (1tan^2x)/sec^2x, integral of cos(2x)Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more

Find The Derivative Of The Given Function Y Tan 2x 1 Cot 2x I Tried Converting The Original Function In Terms Of Sin And Cos But It Was Still Too Complicated To Be Called Simplified

1 Cosx 1 Cosx Tan 2x Secx 1 2prove Brainly In

You ask for the formula of cot(AB) What you mean is the trigonometric identity of that ratio True Start with the well known pythagorean identity sin^2x cos^2x = 1 This is readily derived directly from the definition of the basic trigonometric functions sin and cos and Pythagoras's Theorem Divide both side by cos^2x and we get sin^2x/cos^2x cos^2x/cos^2x = 1/cos^2x tan^2x 1 = sec^2x tan^2x = sec^2x 1 Confirming that the result is an identityTan(x y) = (tan x tan y) / (1 tan x tan y) sin(2x) = 2 sin x cos x cos(2x) = cos ^2 (x) sin ^2 (x) = 2 cos ^2 (x) 1 = 1 2 sin ^2 (x) tan(2x) = 2 tan(x) / (1

Integral Of Tan 2x Cot 2x 2 Calculus 1 Trig Integrals Calculus Mathematics Email Subject Lines

Integral 1 Tan 2 X Sec 2 X Youtube

In this video, I go through a trigonometric proof which is1tan^2=sec^2The proof is fairly straight forward with some common knowledge of trigonometric fuCheck all that apply A cot^2xcsc2x=1 B sin^2xcos^2x=1 C sin^2x = 1 cos^2x D tan^2x = sec^2x1Integration of tan^2x sec^2x/ 1tan^6x dx Ask questions, doubts, problems and we will help you

2

Question Video Differentiating Functions Involving Trigonometric Ratios Using Pythagorean Identities Nagwa

Found 2 solutions by ewatrrr, MathLover1 Answer by ewatrrr () ( Show Source ) You can put this solution on YOUR website! Integral (tan^2x sec^2x)/(1tan^6x)dx Get the answers you need, now!You just studied 17 terms!

Every Day I M Calculatin I D3 Unit Q Pythagorean Identities

Sample Problems Cos 2 X Tan2 X Tan 2 Csc 2 Tan Sec X Tan X Cos X Sin 4 X Cos 4 X 1 2 Cos 2 X Pdf Free Download

 How many solutions of the equation $$\tan^2 x \sec^{10} x 1=0$$ lie in the interval $(0,10)$?Yes, sec 2 x−1=tan 2 x is an identity sec 2 −1=tan 2 x Let us derive the equation We know the identity sin 2 (x)cos 2 (x)=1 ——(i) Dividing throughout the equation by cos 2 (x) We get sin 2 (x)/cos 2 (x) cos 2 (x)/cos 2 (x) = 1/cos 2 (x) We know that sin 2 (x)/cos 2 (x)= tan 2 (x), and cos 2 (x)/cos 2 (x) = 1 So the equation (i) after substituting becomesGet an answer for 'solve tan^2xsecx =1 in the range 0°≤x≤ 360°' and find homework help for other Math questions at eNotes

Tan 2x Tan 2x

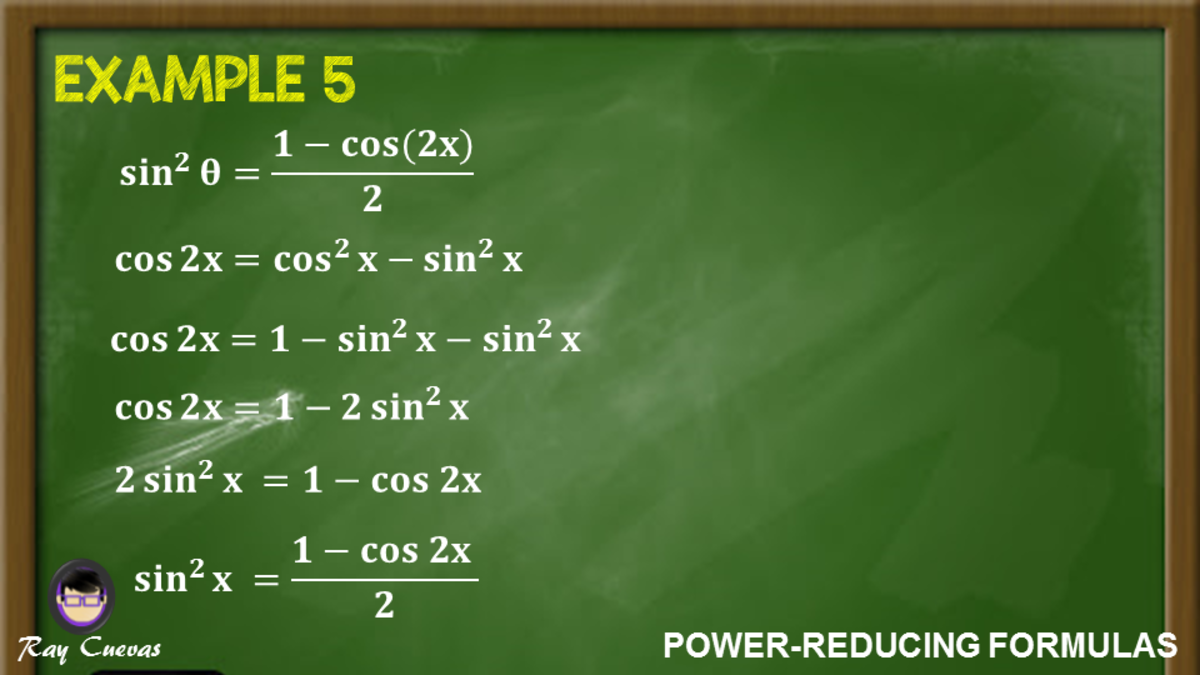
Power Reducing Formulas And How To Use Them With Examples Owlcation

 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queries Students (upto class 102) preparing for All Government Exams, CBSE Board Exam, ICSE Board Exam, State Board Exam, JEE (MainsAdvance) and NEET can ask questions from any subject and get quick answers byQuestion Please help me verify this equation cscx cscx =2sec^2x 1cscx 1cscx yes, the cscx's are over 1cscx and 1cscx Those are fractions So far i have gotten stuck on this porblem i have expanded csc x into 1/sinx in both parts of the left side of The cos2(2x) term is another trigonometric integral with an even power, requiring the powerreducing formula again The cos3(2x) term is a cosine function with an odd power, requiring a substitution as done before We integrate each in turn below ∫cos2(2x) dx = ∫ 1 cos(4x) 2 dx = 1 2 (x 1 4sin(4x)) C

Tan2x Sec2x ただの悪魔の画像

1

 µ • § ¶ ß ‹ › « » < > ≤ ≥ – — ¯ ‾ ¤ ¦ ¨ ¡ ¿ ˆ ˜ ° − ± ÷ ⁄ × ƒ ∫ ∑ ∞ √ ∼ ≅ ≈ ≠ ≡ ∈ ∉ ∋ ∏ ∧ ∨ ¬ ∩ ∪ ∂ ∀ ∃ ∅ ∇ ∗ ∝ ∠ ´ ¸ ª º † ‡ À Á Â Ã Ä Å Æ Ç È É Ê Ë Ì Í Î Ï Ð Ñ Ò Ó Ô Õ Ö Ø Œ Š Ù Ú Û Ü Ý Ÿ Þ à á â ã ä å æ ç è é ê ë ì í î ï ð ñ ò ó ô õ öUse \(\tan^2x=\sec^2x1~(=u^21)\) to replace the leftover tangents \(m\) is even or \(n\) is odd Use either \(1\) or \(2\) (both will work) The power of secant is odd and the power of tangent is even No guideline The integrals \(\ds\int\sec x\,dx\) and \(\ds\int\sec^3 x\,dx\) can usually be looked up, or recalled from memory Example 223Trigonometry Square relation 2 deduction of sec^2x tan^2x = 1with worked out example

Sec 6x Tan 6x 1 2 Tan 2x Sec 2x Important Difficult Trigonometric Identity Youtube

Derivative Of Sec 3 2x

 Get an answer for 'verify (1 tan^2x)/(tan^2x) = csc^2x' and find homework help for other Math questions at eNotesWhat is the value of sin 3x? The identity, as you noted, is tan2x 1 = sec2x, for all values of x Rearranging, you absolutely get tan2x sec2x = 1 So, the original statement is false

2

Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo

For each of the three trigonometric substitutions above we will verify that we can ignore the absolute value in each case when encountering a radical 🔗 For x = asinθ, x = a sin ⁡ θ, the expression √a2 −x2 a 2 − x 2 becomes √a2−x2 = √a2−a2sin2θ= √a2(1−sin2θ)= a√cos2θ= acosθ = acosθ a 2 − x 2 = a 2 − a 2Prove that tan^2x sec^2x=1 Answers 3 Get Other questions on the subject Mathematics Mathematics, 14, klandry0 How long would it take to empty a pond that is 60x50x using a 1000 gallon pump Answers 3 continue Mathematics, 1730, iliketurtures In parallelogram abcd the ratio of ab to bcis 5 3 if theRewrite sec(x) sec ( x) in terms of sines and cosines Rewrite tan(x) tan ( x) in terms of sines and cosines Multiply by the reciprocal of the fraction to divide by 1 cos(x) 1 cos ( x) Write cos(x) cos ( x) as a fraction with denominator 1 1 Cancel the common factor of cos(x) cos ( x)

How Many Can You Derive From First Principles Ppt Download

Http Www Stevejonak Com Test03 Addtional Review Pdf

In this video I go over the proof of the trigonometry identity tan^2(x) 1 = sec^2(x) The proof of this identity is very simple and like many other trig id Which of the following equations are identities? Tan^2 x1=sec^2x So to get 1 on the other side of the equal sign wouldn't it be sec^2xtan^2x=1?Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!1 A water molecule is held together by two single polar covalent bonds False 2 Because oxygen has a greater electronegativity than hydrogen, water molecules are polar with

Tan 2x Tan 2x

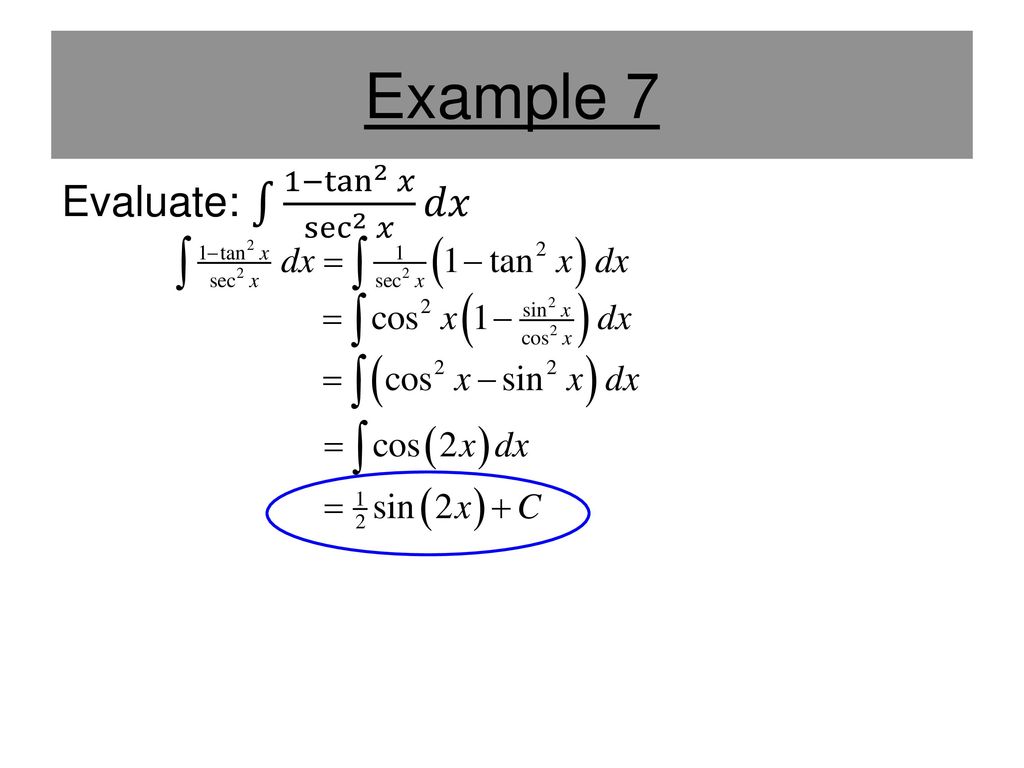
Section 8 3 Trigonometric Integrals Ppt Download

Hi Simplifying the following (sec^2x csc^2x) (tan^2x cot^2x) tan^2x = sec^2x 1 cot^2x = csc^2x 1 (sec^2x csc^2x) (sec^2x 1 csc^2x 1)= 2One to any power is one 1 cos2(x) 1 cos 2 ( x) Because the two sides have been shown to be equivalent, the equation is an identity 1sec2 (x)sin2(x)Trigonometry sin (a/2)= square root of (1cos (a))/2 sin( a 2) = −√ 1 − cos(a) 2 sin ( a 2) = 1 cos ( a) 2 Since the radical is on the right side of the equation, switch the sides so it is on the left side of the equation −√ 1−cos(a) 2 = sin( a 2) 1 cos ( a) 2 = sin ( a 2)

Orion Math Iastate Edu Miriamc 8 2 Notes166 Sp17 Pdf

Verifying A Trigonometric Identity Tan 2 X 1 Sec X Sec X Youtube

If we are dealing with 'real' numbers, the sine function returns a value in the range 1, 1, thus, depending on what x is, the answer will be a number whose absolute value is less than or equal to one If you aren'tLet y = `tan^(1) (1cosx)/(sin x)` Then `=> y = tan^(1) ((2cos^2 x/2)/(2sin x/2,cos x/2))` `=> y = tan^1 (cot x/2)` `=> y = tan^1 {tan (pi/2 x/2)}`Now up your study game with Learn mode

Lim X To 0 2 Tan X 2 Sin X X 2 Sin X Without L Hopital S Rule How Is My Procedure Wrong Mathematics Stack Exchange

Trigonometry Identity Tan 2 X 1 Sec 2 X Youtube

Simplify and write the trigonometric expression in terms of sine and cosine (2tan^2x / sec^2x) 1 = (f (x))^2 f (x) =Free trigonometric identities list trigonometric identities by request stepbystep

Chapter 2 Answers

Sec 6x Tan 6x 1 3 Tan 2x Sec 2x Brainly In

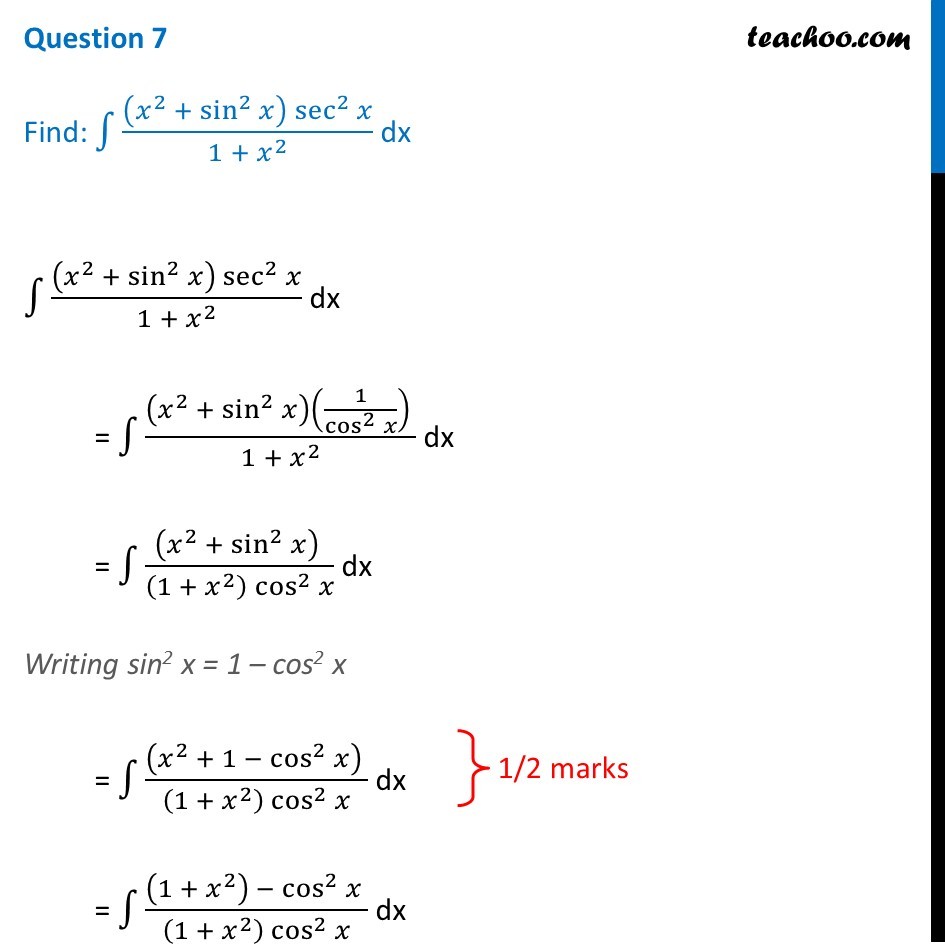
Find Integral X 2 Sin 2 X Sec 2 X 1 X 2 Teachoo

Prove That Sec8x 1 Sec4x 1 Tan8x Tan2x

Cos 2x

Calameo Diapositivas Lenny Vanegas

Answered Let F X Tan 2x Which Of The Bartleby

Sin2x Cos2x 1 Proof

2

Tan 2x 2tanx 5dy Dx 2 1 Tanx Sec 2x Differential Equations Brainly In

Question 34 Ssssd Maths Integrals Meritnation Com

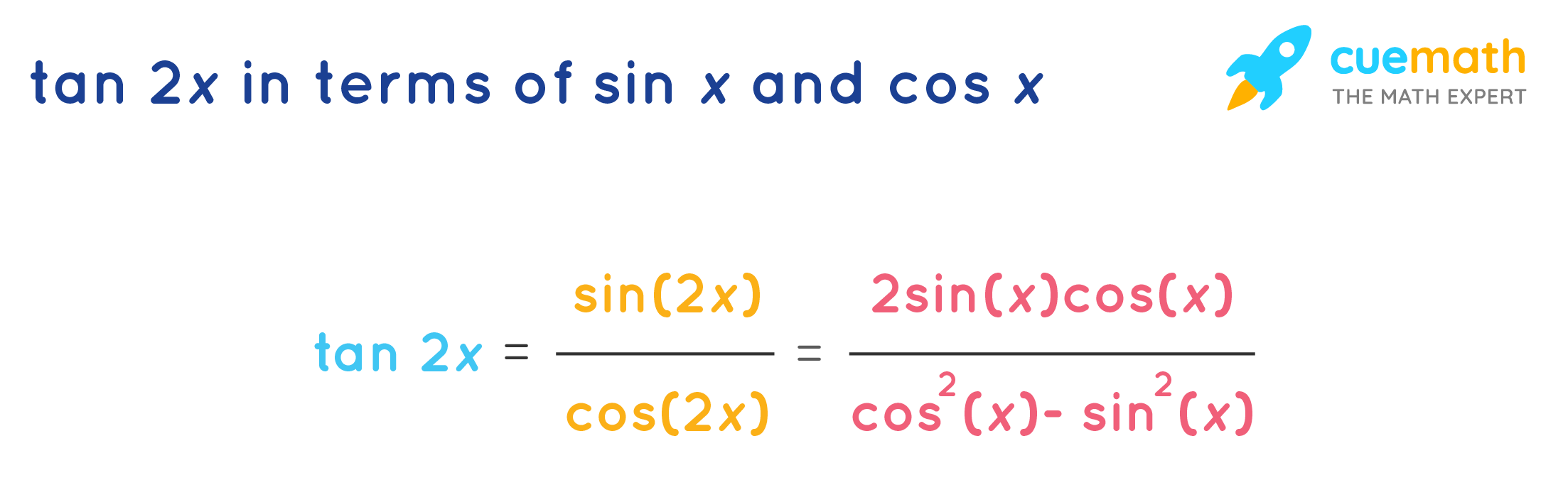
Tan 2x Formula What Is Tan 2x Formula Examples

How To Integrate Secx A Btanx Quora

2

Answered If F X Tan 2x Then F X 3d Bartleby

If Y Tan 2x Sec 2x Find Dy Dx Brainly In

1 Sin 2x Tan 2x Sec Sec 2 X Csc 2x 1 1 C Gauthmath

How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Socratic

The Derivative Of Sec2x Derivativeit

Differential Equation D 2 1 Y E X Sec 2 X Tan X Mathematics Stack Exchange

2

How Many Can You Derive From First Principles Ppt Download

Differentiate The Following From First Principle Tan 2x 1

Yorkshiremathstutor Com Wp Content Uploads 19 02 8 Differentiation Answers Pdf

2

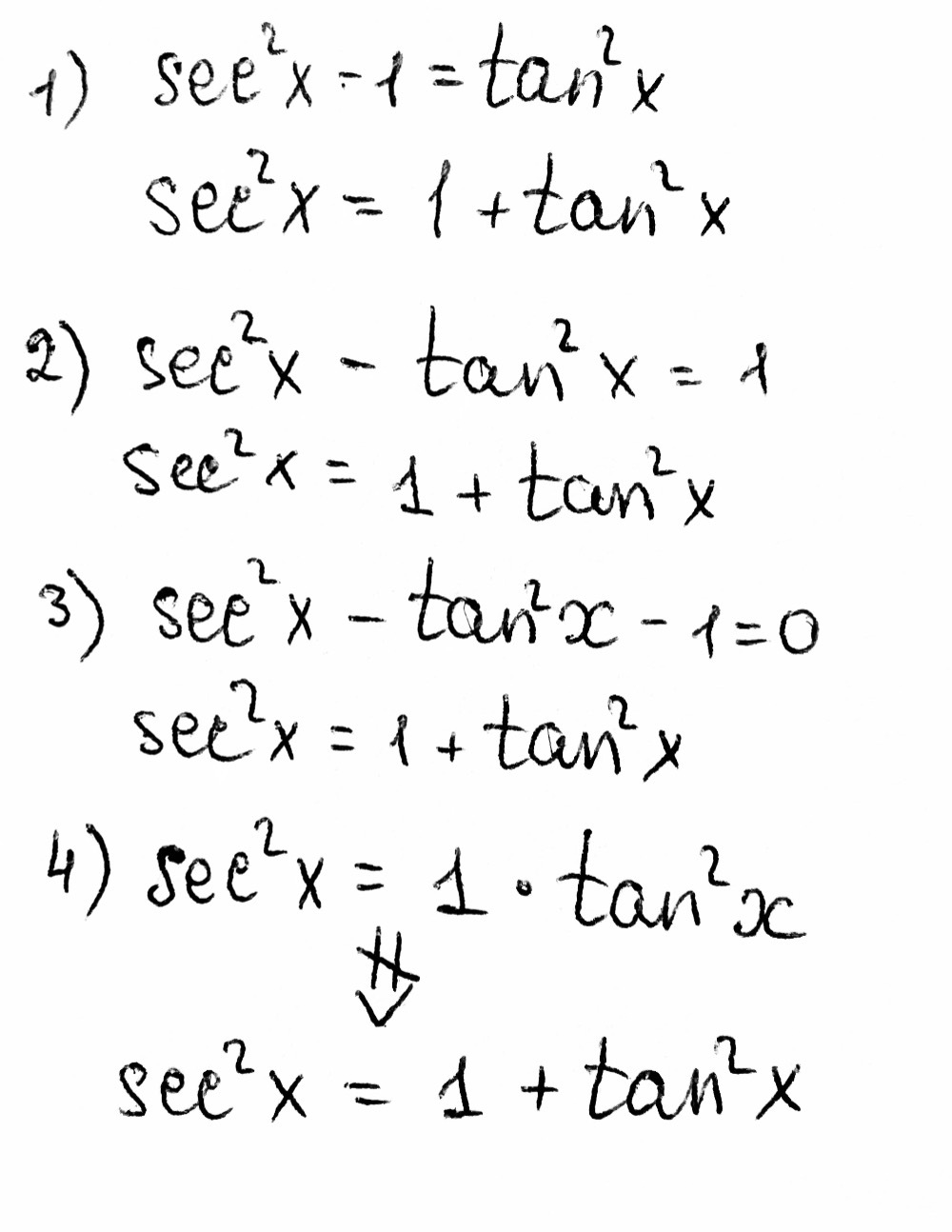
Which One Is Not Equivalent To Sec2x 1 Tan2x Sec Gauthmath

Solutions To Odd Number Exercises Lo Y Sec 2 Period

Trig Identity Sec2x Minus Tan2x T10 Youtube

How Do You Solve 1 Tan 2x 6 2sec 2x Socratic

2

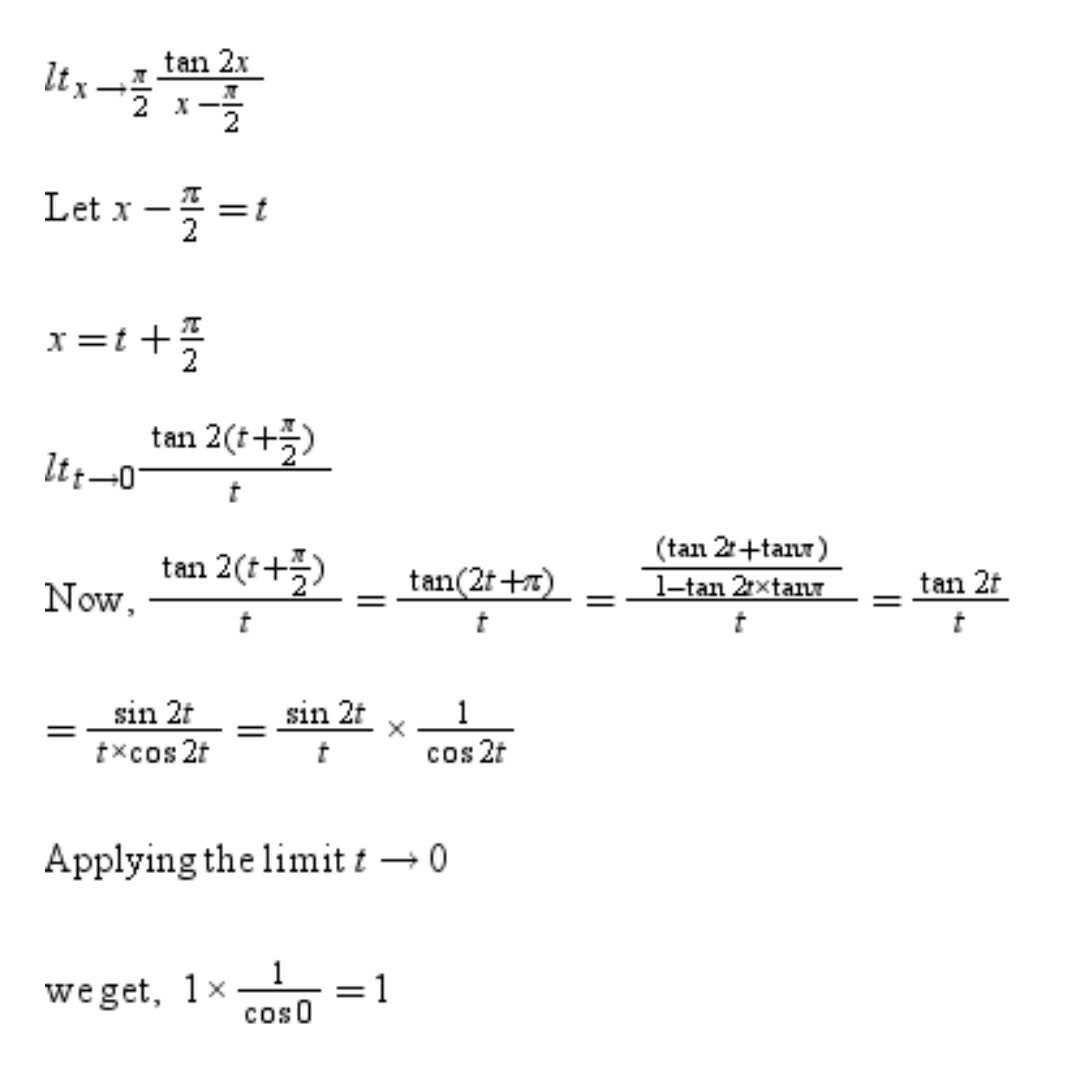
Lim X Rightarrow Frac Pi 2 Frac Tan 2x Scholr

Ppt Analytic Trig Powerpoint Presentation Free Download Id

2

2

1

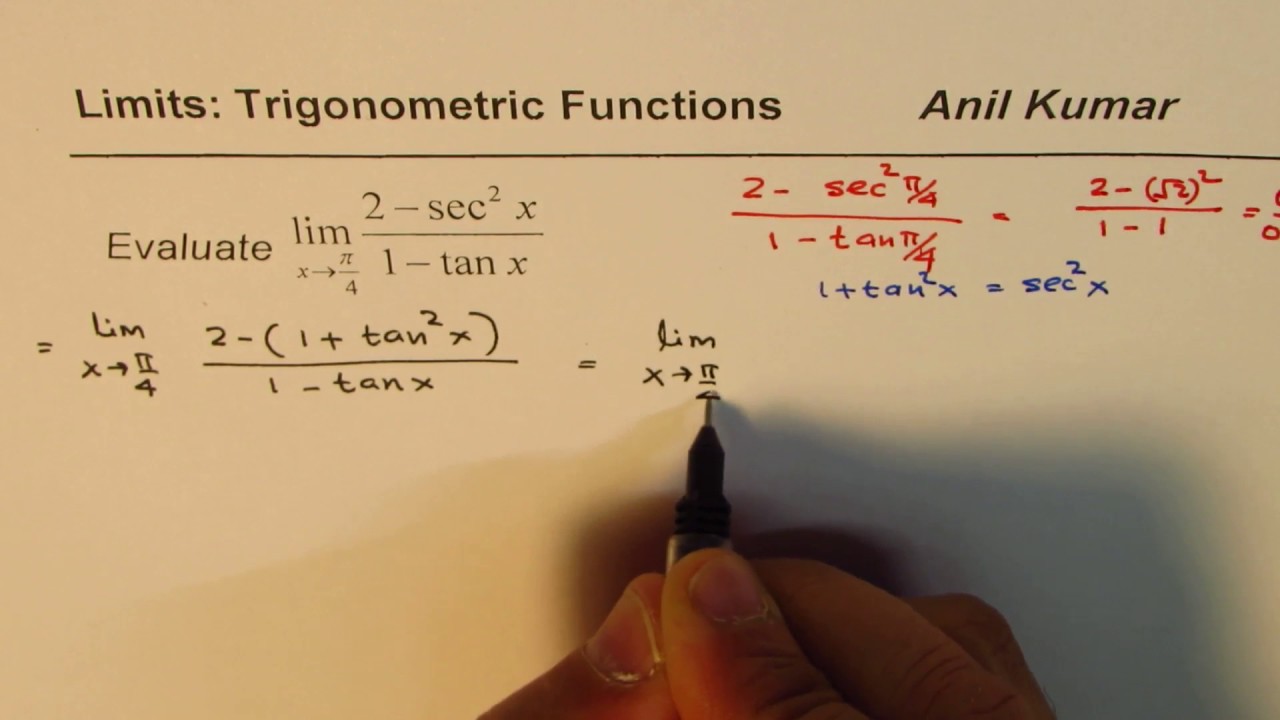
Limit Trigonometric Function 2 Sec 2x 1 Tan X Youtube

0 21 Solve By Inversion Method Y 1 0 22 Find The General Solution Of

How Do You Prove Tan 2x Secx 1 1 Secx Socratic

How Do You Simplify Sec 4x Tan 4x Sec 2x Tan 2x Socratic

Which Of The Following Are Trigonometric Identitie Gauthmath

Solved Evaluate The Derivatives Of The Following Functions With Respect To X And Simplify The Results 2 Points Each 1 Y 3 X 6 2 Y 2x 1 Course Hero

A Solution Of The Equation 1 Tan X 1 Tan X Sec 2x 2 Tan 2 0 Where X Lies In The Interval Pi2 Pi2 Is Given By

Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo

Trig Identity Tan X 1 Tan 2 X Sec X Cos X Csc X Sin X Proved From Both Sides Youtube

Hs Trig Math Help Solving Trig Equations But I Don T Know How To Move Forward After Factoring Homeworkhelp

Evaluate Lim Xrightarrow Frac Pi 2 Frac Tan 2x X Frac Pi 2 Orevaluate Lim Xrightarrow 3 Frac X 4 81 2x 2 5x 3 Snapsolve

I Int Sec 2x Tanxsqrt Tan 2x 1 Dx

Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo

Answered 3 1 Tanx Tan 2x Sec 2x S Bartleby

Int Tan 2x Secx 1 Dx Youtube

Sample Problems Cos 2 X Tan2 X Tan 2 Csc 2 Tan Sec X Tan X Cos X Sin 4 X Cos 4 X 1 2 Cos 2 X Pdf Free Download

2

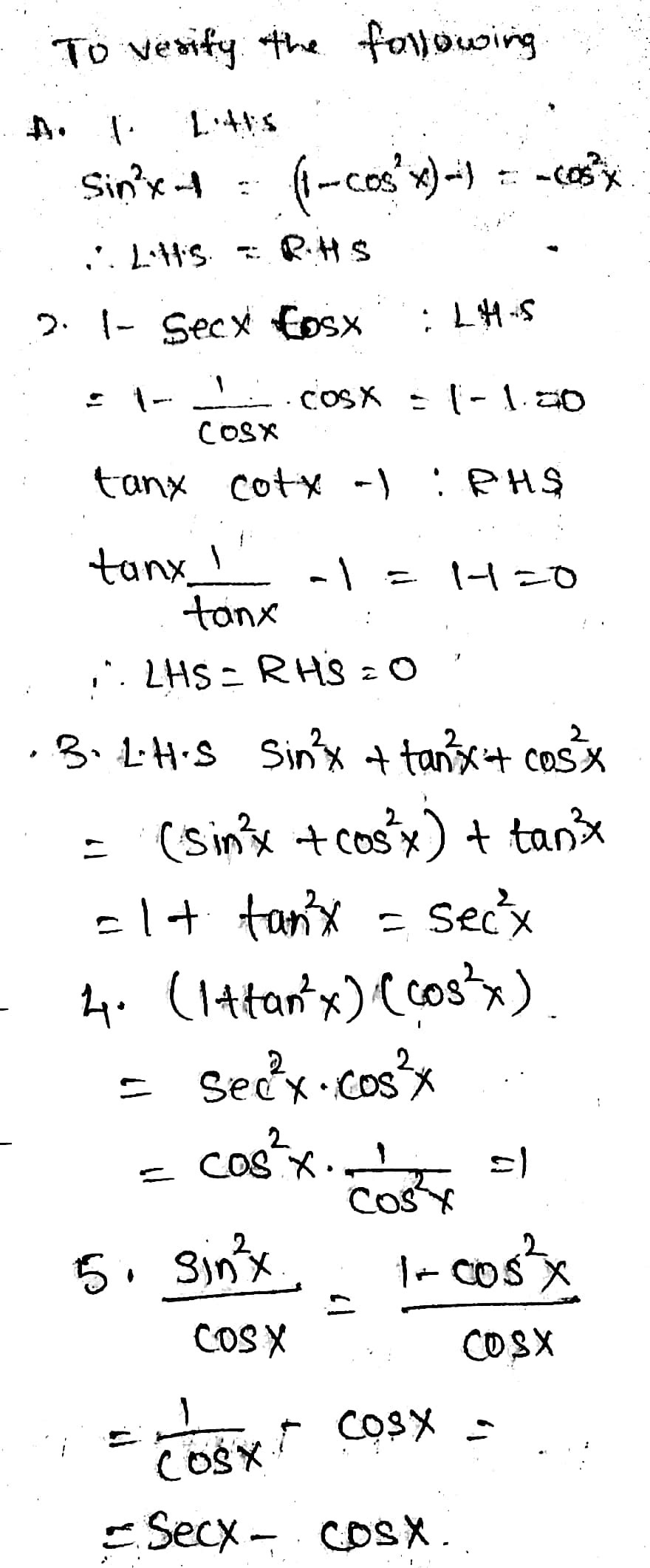
Directions Verify The Following Trigonometric Id Gauthmath

Sec 6x Tan 6x 3sec 2xtan 2x 1 Solution

Tan 2x Formula What Is Tan 2x Formula Examples

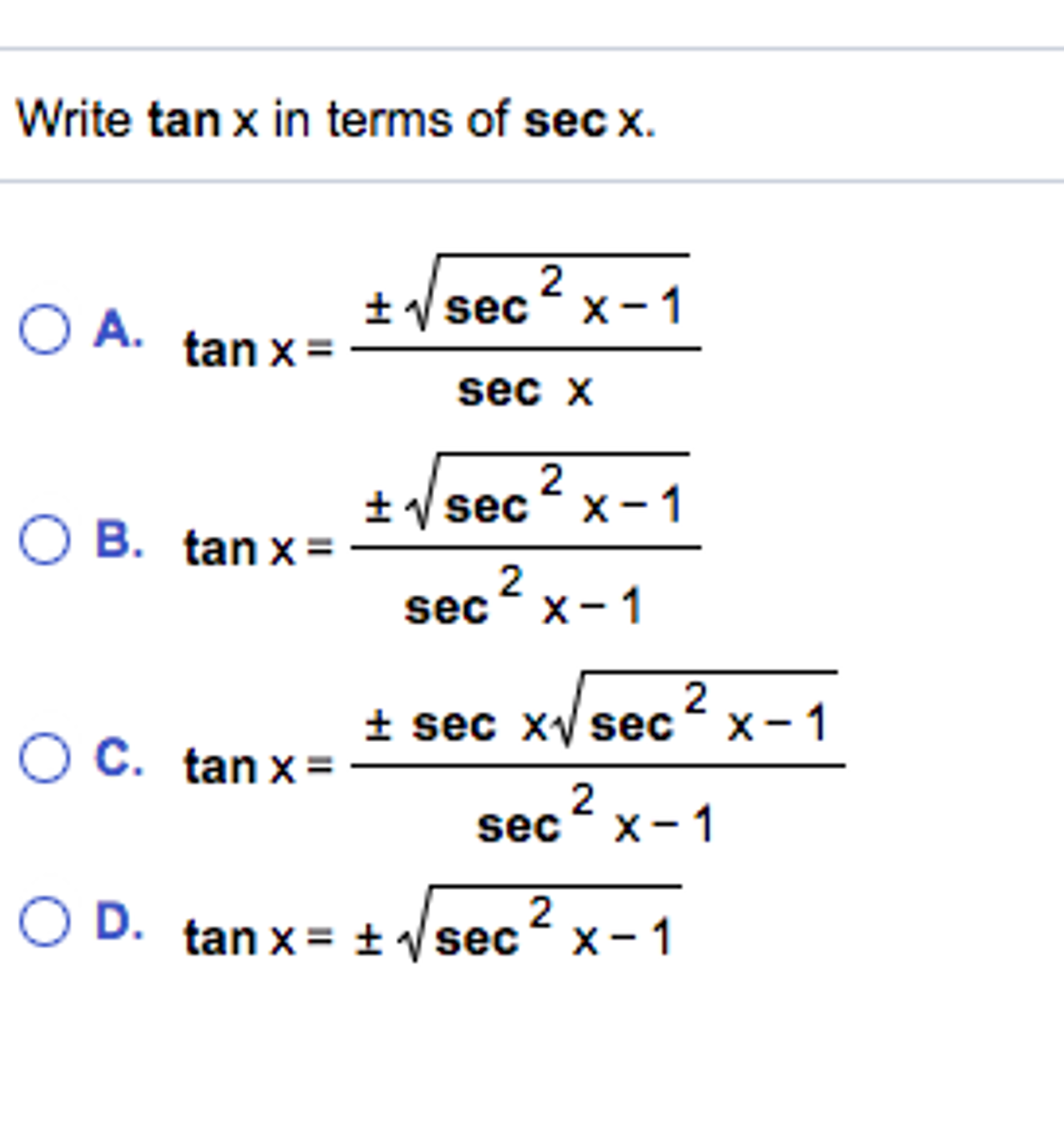
Write Tan X In Terms Of Sec X Tan X Plusminus Chegg Com

Sec 2 2x 1 Tan 2x Snapsolve

If Tan 2 X Sec X A 0 Has Atleast One Solution Then Complete Set Of V Askiitians

Integration Trig Identities Ppt Download

Ppt 5 1 Fundamental Trig Identities Powerpoint Presentation Free Download Id

2

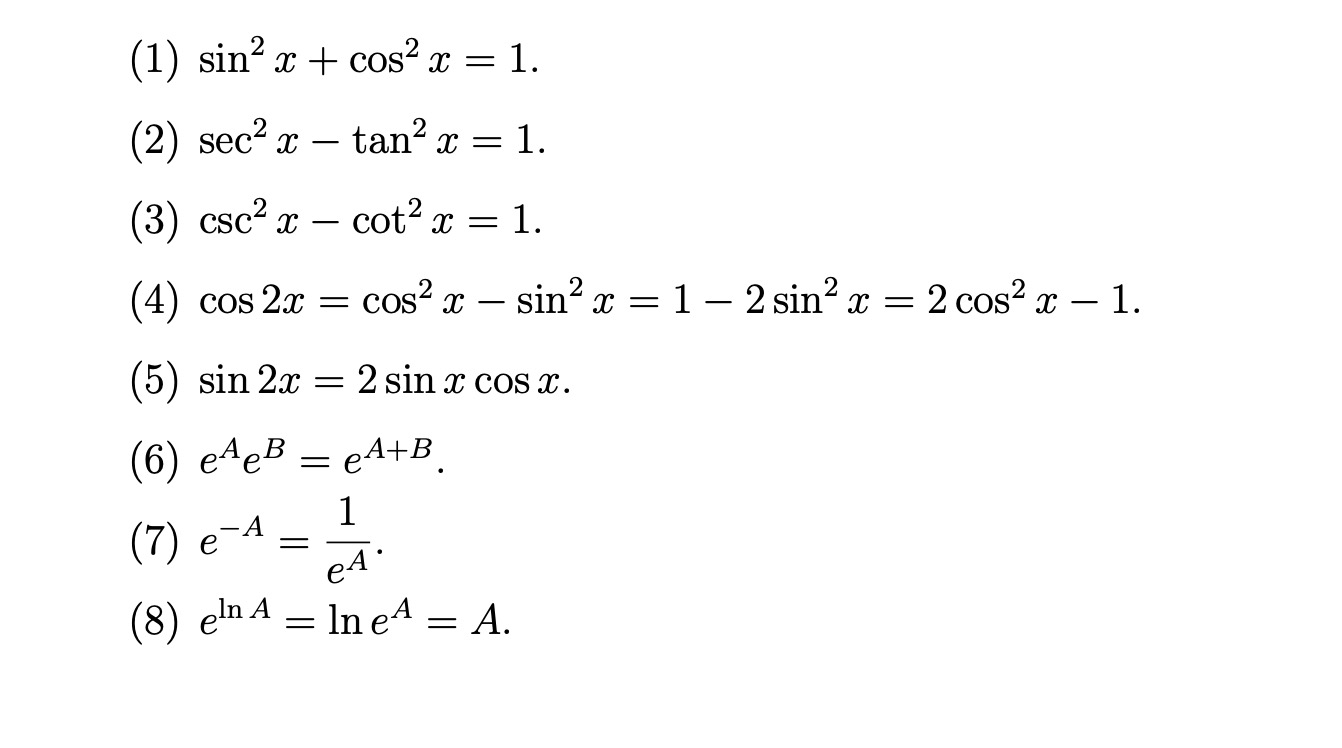
Here Is A List Of Identities Involving Trigonometric Chegg Com

Evaluate The Following Integral Sec 2x Tan 2x Dx Sec Chegg Com

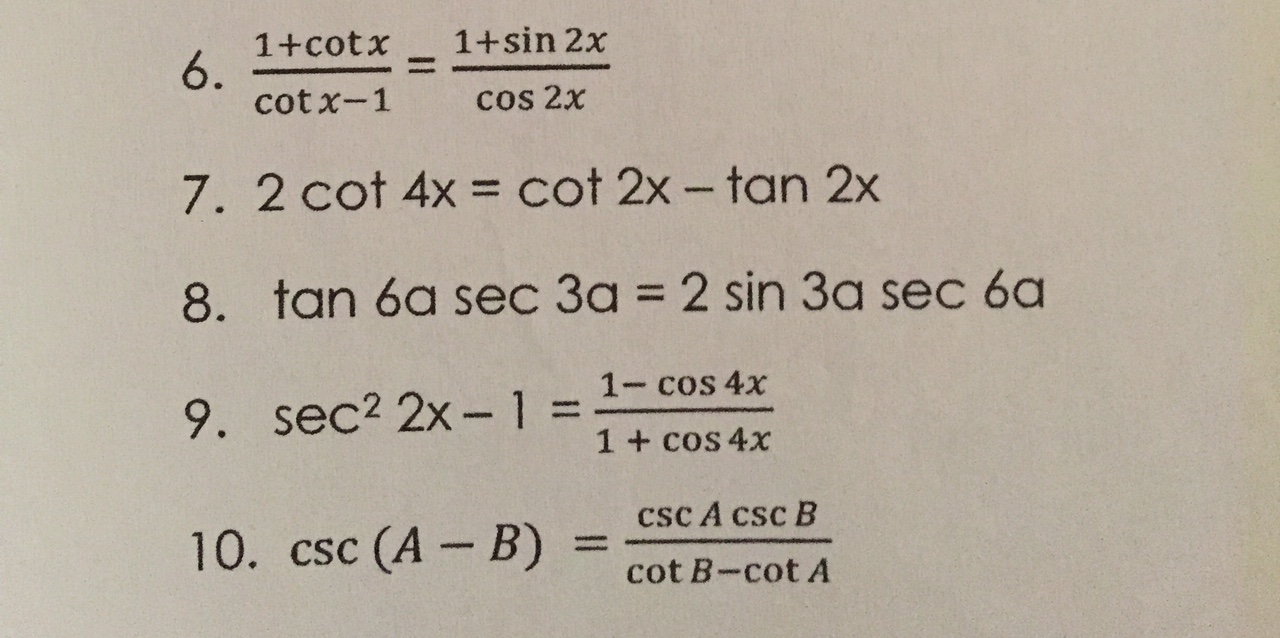
1 Cotx 1 Sin 2x 6 Cotx 1 Cos 2x 7 2 Cot 4x Cot 2x Chegg Com

Examples Int Tan 2x Sec 4x Dx

1 Cosx 1 Cosx Tan 2x Secx 1 2 Brainly In

What Is The Derivative Of Tan 1 Sec X Tan X Quora

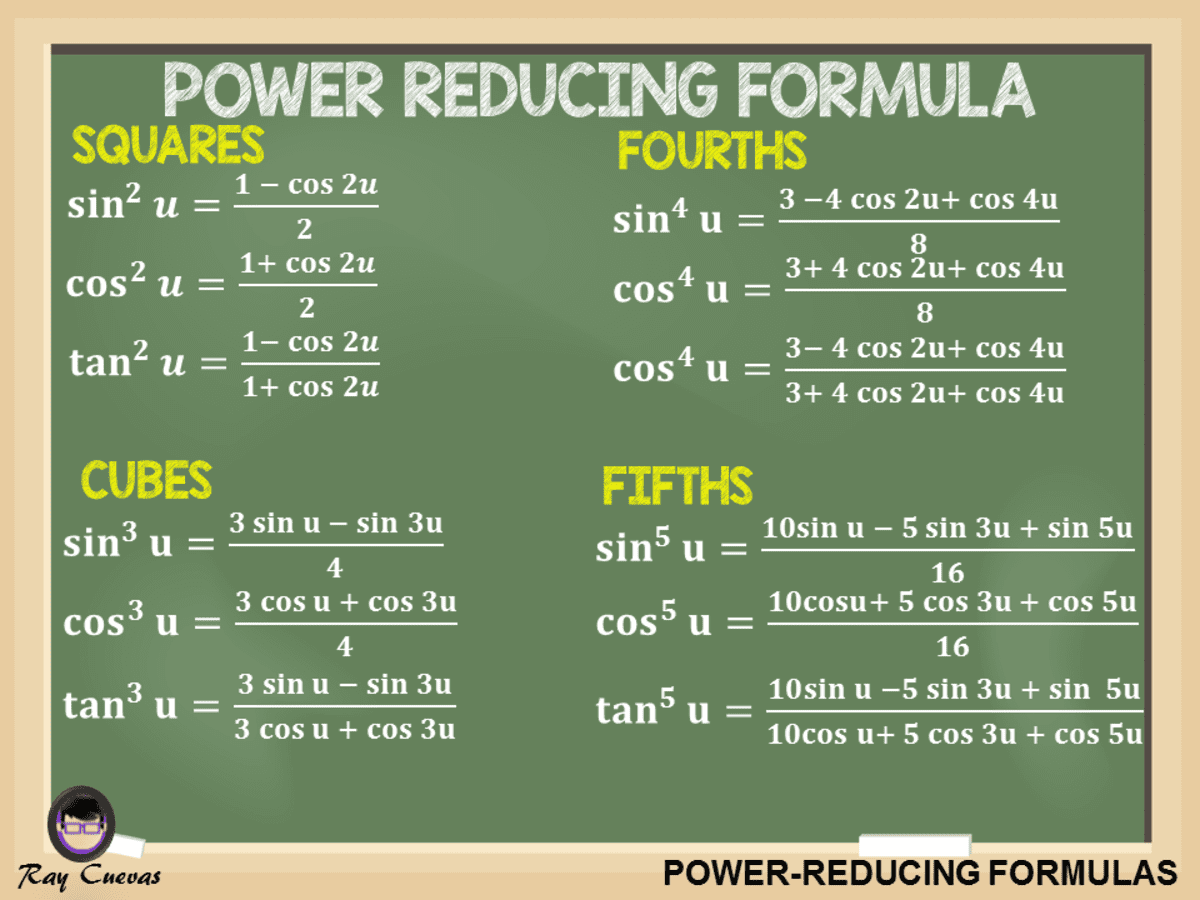
Power Reducing Formulas And How To Use Them With Examples Owlcation

1

Int Tanx Sec 2 X 1 Tan 2 X Dx

Http Www Math Brown Edu Tomg Formulas Pdf

1

2

Question 1 Integrate The Following Function Sec 2x Chegg Com

Solved Prove The Following Trig Identity Sec 2 X 2secx Cosx Cos 2 X Tan 2 X Sin 2 X Course Hero

Http Www Wssd Org Cms Lib02 Pa Centricity Domain 140 1 4 1 6 Pdf

How Does One Verify Cos 2x Sin 2x 1 Tan 2x Cos 2x Socratic

How Do You Integrate 1 Tan2x Sec2x Dx Socratic

The Value Of Int Tan 3 2x Sec 2x Dx Is Equal To

How Do You Prove Tan 2x Secx 1 1 Secx Socratic

Incoming Term: 5.25064634,